What 1% of Overround Worth?
/All You Ever Wanted to Know About Favourite-Longshot Bias ...
/Previously, on at least a few occasions, I've looked at the topic of the Favourite-Longshot Bias and whether or not it exists in the TAB Sportsbet wagering markets for AFL.
A Favourite-Longshot Bias (FLB) is said to exist when favourites win at a rate in excess of their price-implied probability and longshots win at a rate less than their price-implied probability. So if, for example, teams priced at $10 - ignoring the vig for now - win at a rate of just 1 time in 15, this would be evidence for a bias against longshots. In addition, if teams priced at $1.10 won, say, 99% of the time, this would be evidence for a bias towards favourites.
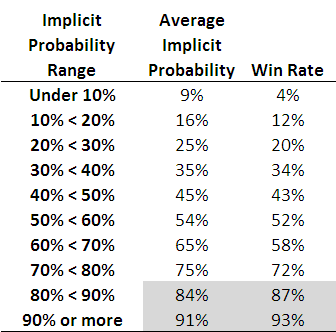
When I've considered this topic in the past I've generally produced tables such as the following, which are highly suggestive of the existence of such an FLB.
Each row of this table, which is based on all games from 2006 to the present, corresponds to the results for teams with price-implied probabilities in a given range. The first row, for example, is for all those teams whose price-implied probability was less than 10%. This equates, roughly, to teams priced at $9.50 or more. The average implied probability for these teams has been 9%, yet they've won at a rate of only 4%, less than one-half of their 'expected' rate of victory.
As you move down the table you need to arrive at the second-last row before you come to one where the win rate exceed the expected rate (ie the average implied probability). That's fairly compelling evidence for an FLB.
This empirical analysis is interesting as far as it goes, but we need a more rigorous statistical approach if we're to take it much further. And heck, one of the things I do for a living is build statistical models, so you'd think that by now I might have thrown such a model at the topic ...
A bit of poking around on the net uncovered this paper which proposes an eminently suitable modelling approach, using what are called conditional logit models.
In this formulation we seek to explain a team's winning rate purely as a function of (the natural log of) its price-implied probability. There's only one parameter to fit in such a model and its value tells us whether or not there's evidence for an FLB: if it's greater than 1 then there is evidence for an FLB, and the larger it is the more pronounced is the bias.
When we fit this model to the data for the period 2006 to 2010 the fitted value of the parameter is 1.06, which provides evidence for a moderate level of FLB. The following table gives you some idea of the size and nature of the bias.
The first row applies to those teams whose price-implied probability of victory is 10%. A fair-value price for such teams would be $10 but, with a 6% vig applied, these teams would carry a market price of around $9.40. The modelled win rate for these teams is just 9%, which is slightly less than their implied probability. So, even if you were able to bet on these teams at their fair-value price of $10, you'd lose money in the long run. Because, instead, you can only bet on them at $9.40 or thereabouts, in reality you lose even more - about 16c in the dollar, as the last column shows.
We need to move all the way down to the row for teams with 60% implied probabilities before we reach a row where the modelled win rate exceeds the implied probability. The excess is not, regrettably, enough to overcome the vig, which is why the rightmost entry for this row is also negative - as, indeed, it is for every other row underneath the 60% row.
Conclusion: there has been an FLB on the TAB Sportsbet market for AFL across the period 2006-2010, but it hasn't been generally exploitable (at least to level-stake wagering).
The modelling approach I've adopted also allows us to consider subsets of the data to see if there's any evidence for an FLB in those subsets.
I've looked firstly at the evidence for FLB considering just one season at a time, then considering only particular rounds across the five seasons.
So, there is evidence for an FLB for every season except 2007. For that season there's evidence of a reverse FLB, which means that longshots won more often than they were expected to and favourites won less often. In fact, in that season, the modelled success rate of teams with implied probabilities of 20% or less was sufficiently high to overcome the vig and make wagering on them a profitable strategy.
That year aside, 2010 has been the year with the smallest FLB. One way to interpret this is as evidence for an increasing level of sophistication in the TAB Sportsbet wagering market, from punters or the bookie, or both. Let's hope not.
Turning next to a consideration of portions of the season, we can see that there's tended to be a very mild reverse FLB through rounds 1 to 6, a mild to strong FLB across rounds 7 to 16, a mild reverse FLB for the last 6 rounds of the season and a huge FLB in the finals. There's a reminder in that for all punters: longshots rarely win finals.
Lastly, I considered a few more subsets, and found:
- No evidence of an FLB in games that are interstate clashes (fitted parameter = 0.994)
- Mild evidence of an FLB in games that are not interstate clashes (fitted parameter = 1.03)
- Mild to moderate evidence of an FLB in games where there is a home team (fitted parameter = 1.07)
- Mild to moderate evidence of a reverse FLB in games where there is no home team (fitted parameter = 0.945)
FLB: done.
Divining the Bookie Mind: Singularly Difficult
/It's fun this time of year to mine the posted TAB Sportsbet markets in an attempt to glean what their bookie is thinking about the relative chances of the teams in each of the four possible Grand Final pairings.
Three markets provide us with the relevant information: those for each of the two Preliminary Finals, and that for the Flag.
From these markets we can deduce the following about the TAB Sportsbet bookie's current beliefs (making my standard assumption that the overround on each competitor in a contest is the same, which should be fairly safe given the range of probabilities that we're facing with the possible exception of the Dogs in the Flag market):
- The probability of Collingwood defeating Geelong this week is 52%
- The probability of St Kilda defeating the Dogs this week is 75%
- The probability of Collingwood winning the Flag is about 34%
- The probability of Geelong winning the Flag is about 32%
- The probability of St Kilda winning the Flag is about 27%
- The probability of the Western Bulldogs winning the Flag is about 6%
(Strictly speaking, the last probability is redundant since it's implied by the three before it.)
What I'd like to know is what these explicit probabilities imply about the implicit probabilities that the TAB Sportsbet bookie holds for each of the four possible Grand Final matchups - that is for the probability that the Pies beat the Dogs if those two teams meet in the Grand Final; that the Pies beat the Saints if, instead, that pair meet; and so on for the two matchups involving the Cats and the Dogs, and the Cats and the Saints.
It turns out that the six probabilities listed above are insufficient to determine a unique solution for the four Grand Final probabilities I'm after - in mathematical terms, the relevant system that we need to solve is singular.
That system is (approximately) the following four equations, which we can construct on the basis of the six known probabilities and the mechanics of which team plays which other team this week and, depending on those results, in the Grand Final:
- 52% x Pr(Pies beat Dogs) + 48% x Pr(Cats beat Dogs) = 76%
- 52% x Pr(Pies beat Saints) + 48% x Pr(Cats beat Saints) = 63.5%
- 75% x Pr(Pies beat Saints) + 25% x Pr(Pies beat Dogs) = 66%
- 75% x Pr(Cats beat Saints) + 25% x Pr(Cats beat Dogs) = 67.5%
(If you've a mathematical bent you'll readily spot the reason for the singularity in this system of equations: the coefficients in every equation sum to 1, as they must since they're complementary probabilities.)
Whilst there's not a single solution to those four equations - actually there's an infinite number of them, so you'll be relieved to know that I won't be listing them all here - the fact that probabilities must lie between 0 and 1 puts constraints on the set of feasible solutions and allows us to bound the four probabilities we're after.
So, I can assert that, as far as the TAB Sportsbet bookie is concerned:
- The probability that Collingwood would beat St Kilda if that were the Grand Final matchup - Pr(Pies beats Saints) in the above - is between about 55% and 70%
- The probability that Collingwood would beat the Dogs if that were the Grand Final matchup is higher than 54% and, of course, less than or equal to 100%.
- The probability that Geelong would beat St Kilda if that were the Grand Final matchup is between 57% and 73%
- The probability that Geelong would beat the Dogs if that were the Grand Final matchup is higher than 50.5% and less than or equal to 100%.
One straightforward implication of these assertions is that the TAB Sportsbet bookie currently believes the winner of the Pies v Cats game on Friday night will start as favourite for the Grand Final. That's an interesting conclusion when you recall that the Saints beat the Cats in week 1 of the Finals.
We can be far more definitive about the four probabilities if we're willing to set the value of any one of them, as this then uniquely defines the other three.
So, let's assume that the bookie thinks that the probability of Collingwood defeating the Dogs if those two make the Grand Final is 80%. Given that, we can say that the bookie must also believe that:
- The probability that Collingwood would beat St Kilda if that were the Grand Final matchup is about 61%.
- The probability that Geelong would beat St Kilda if that were the Grand Final matchup, is about 66%.
- The probability that Geelong would beat the Dogs if that were the Grand Final matchup is higher than 72%.
Together, that forms a plausible set of probabilities, I'd suggest, although the Geelong v St Kilda probability is higher than I'd have guessed. The only way to reduce that probability though is to also reduce the probability of the Pies beating the Dogs.
If you want to come up with your own rough numbers, choose your own probability for the Pies v Dogs matchup and then adjust the other three probabilities using the four equations above or using the following approximation:
For every 5% that you add to the Pies v Dogs probability:
- subtract 1.5% from the Pies v Saints probability
- add 2% to the Cats v Saints probability, and
- subtract 5.5% from the Cats v Dogs probability
If you decide to reduce rather than increase the probability for the Pies v Dogs game then move the other three probabilities in the direction opposite to that prescribed in the above. Also, remember that you can't drop the Pies v Dogs probability below 55% nor raise it above 100% (no matter how much better than the Dogs you think the Pies are, the laws of probability must still be obeyed.)
Alternatively, you can just use the table below if you're happy to deal only in 5% increments of the Pies v Dogs probability. Each row corresponds to a set of the four probabilities that is consistent with the TAB Sportsbet markets as they currently stand.
I've highlighted the four rows in the table that I think are the ones most likely to match the actual beliefs of the TAB Sportsbet bookie. That narrows each of the four probabilities into a 5-15% range.
At the foot of the table I've then converted these probability ranges into equivalent fair-value price ranges. You should take about 5% off these prices if you want to obtain likely market prices.
Line Betting : A Codicil
/A Line Betting Enigma
/The Importance of a Team's Recent Form: What Bookies (and MARS) Think
/Predicting Head-to-Head Market Prices
/The Relationship Between Head-to-Head Price and Points Start
/What Do Bookies Know That We Don't?
/What Price the Saints to Beat the Cats in the GF?
/If the Grand Final were to be played this weekend, what prices would be on offer?
We can answer this question for the TAB Sportsbet bookie using his prices for this week's games, his prices for the Flag market and a little knowledge of probability.
Consider, for example, what must happen for the Saints to win the flag. They must beat the Dogs this weekend and then beat whichever of the Cats or the Pies wins the other Preliminary Final. So, there are two mutually exclusive ways for them to win the Flag.
In terms of probabilities, we can write this as:
Prob(St Kilda Wins Flag) =
Prob(St Kilda Beats Bulldogs) x Prob (Geelong Beats Collingwood) x Prob(St Kilda Beats Geelong) +
Prob(St Kilda Beats Bulldogs) x Prob (Collingwood Beats Geelong) x Prob(St Kilda Beats Collingwood)
We can write three more equations like this, one for each of the other three Preliminary Finalists.
Now if we assume that the bookie's overround has been applied to each team equally then we can, firstly, calculate the bookie's probability of each team winning the Flag based on the current Flag market prices which are St Kilda $2.40; Geelong $2.50; Collingwood $5.50; and Bulldogs $7.50.
If we do this, we obtain:
- Prob(St Kilda Wins Flag) = 36.8%
- Prob(Geelong Wins Flag) = 35.3%
- Prob(Collingwood Wins Flag) = 16.1%
- Prob(Bulldogs Win Flag) = 11.8%
Next, from the current head-to-head prices for this week's games, again assuming equally applied overround, we can calculate the following probabilities:
- Prob(St Kilda Beats Bulldogs) = 70.3%
- Prob(Geelong Beats Collingwood) = 67.8%
Armed with those probabilities and the four equations of the form of the one above in bold we come up with a set of four equations in four unknowns, the unknowns being the implicit bookie probabilities for all the possible Grand Final matchups.
To lapse into the technical side of things for a second, we have a system of equations Ax = b that we want to solve for x. But, it turns out, the A matrix is rank-deficient. Mathematically this means that there are an infinite number of solutions for x; practically it means that we need to define one of the probabilities in x and we can then solve for the remainder.
Which probability should we choose?
I feel most confident about setting a probability - or a range of probabilities - for a St Kilda v Geelong Grand Final. St Kilda surely would be slight favourites, so let's solve the equations for Prob(St Kilda Beats Geelong) equal to 51% to 57%.
Each column of the table above provides a different solution and is obtained by setting the probability in the top row and then solving the equations to obtain the remaining probabilities.
The solutions in the first 5 columns all have the same characteristic, namely that the Saints are considered more likely to beat the Cats than they are to beat the Pies. To steal a line from Get Smart, I find that hard to believe, Max.
Inevitably then we're drawn to the last two columns of the table, which I've shaded in gray. Either of these solutions, I'd contend, are valid possibilities for the TAB Sportsbet bookie's true current Grand Final matchup probabilities.
If we turn these probabilities into prices, add a 6.5% overround to each, and then round up or down as appropriate, this gives us the following Grand Final matchup prices.
St Kilda v Geelong
- $1.80/$1.95 or $1.85/$1.90
St Kilda v Collingwood
- $1.75/$2.00 or $1.70/$2.10
Geelong v Bulldogs
- $1.50/$2.45 or $1.60/$2.30
Collingwood v Bulldogs
- $1.65/$2.20 or $1.50/$2.45
Are Footy HAMs Normal?
/Okay, this is probably going to be a long blog so you might want to make yourself comfortable.
For some time now I've been wondering about the statistical properties of the Handicap-Adjusted Margin (HAM). Does it, for example, follow a normal distribution with zero mean?
Well firstly we need to deal with the definition of the term HAM, for which there is - at least - two logical definitions.
The first definition, which is the one I usually use, is calculated from the Home Team perspective and is Home Team Score - Away Team Score + Home Team's Handicap (where the Handicap is negative if the Home Team is giving start and positive otherwise). Let's call this Home HAM.
As an example, if the Home Team wins 112 to 80 and was giving 20.5 points start, then Home HAM is 112-80-20.5 = +11.5 points, meaning that the Home Team won by 11.5 points on handicap.
The other approach defines HAM in terms of the Favourite Team and is Favourite Team Score - Underdog Team Score + Favourite Team's Handicap (where the Handicap is always negative as, by definition the Favourite Team is giving start). Let's call this Favourite HAM.
So, if the Favourite Team wins 82 to 75 and was giving 15.5 points start, then Favourite HAM is 82-75-15.5 = -7.5 points, meaning that the Favourite Team lost by 7.5 points on handicap.
Home HAM will be the same as Favourite HAM if the Home Team is Favourite. Otherwise Home HAM and Favourite HAM will have opposite signs.
There is one other definitional detail we need to deal with and that is which handicap to use. Each week a number of betting shops publish line markets and they often differ in the starts and the prices offered for each team. For this blog I'm going to use TAB Sportsbet's handicap markets.
TAB Sportsbet Handicap markets work by offering even money odds (less the vigorish) on both teams, with one team receiving start and the other offering that same start. The only exception to this is when the teams are fairly evenly matched in which case the start is fixed at 6.5 points and the prices varied away from even money as required. So, for example, we might see Essendon +6.5 points against Carlton but priced at $1.70 reflecting the fact that 6.5 points makes Essendon in the bookie's opinion more likely to win on handicap than to lose. Games such as this are problematic for the current analysis because the 'true' handicap is not 6.5 points but is instead something less than 6.5 points. Including these games would bias the analysis - and adjusting the start is too complex - so we'll exclude them.
So, the question now becomes is HAM Home, defined as above and using the TAB Sportsbet handicap and excluding games with 6.5 points start or fewer, normally distributed with zero mean? Similarly, is HAM Favourite so distributed?
We should expect HAM Home and HAM Favourite to have zero means because, if they don't it suggests that the Sportsbet bookie has a bias towards or against Home teams of Favourites. And, as we know, in gambling, bias is often financially exploitable.
There's no particular reason to believe that HAM Home and HAM Favourite should follow a normal distribution, however, apart from the startling ubiquity of that distribution across a range of phenomena.
Consider first the issue of zero means.
The following table provides information about Home HAMs for seasons 2006 to 2008 combined, for season 2009, and for seasons 2006 to 2009. I've isolated this season because, as we'll see, it's been a slightly unusual season for handicap betting.
Each row of this table aggregates the results for different ranges of Home Team handicaps. The first row looks at those games where the Home Team was offering start of 30.5 points or more. In these games, of which there were 53 across seasons 2006 to 2008, the average Home HAM was 1.1 and the standard deviation of the Home HAMs was 39.7. In season 2009 there have been 17 such games for which the average Home HAM has been 14.7 and the standard deviation of the Home HAMs has been 29.1.
The asterisk next to the 14.7 average denotes that this average is statistically significantly different from zero at the 10% level (using a two-tailed test). Looking at other rows you'll see there are a handful more asterisks, most notably two against the 12.5 to 17.5 points row for season 2009 denoting that the average Home HAM of 32.0 is significant at the 5% level (though it is based on only 8 games).
At the foot of the table you can see that the overall average Home HAM across seasons 2006 to 2008 was, as we expected approximately zero. Casting an eye down the column of standard deviations for these same seasons suggests that these are broadly independent of the Home Team handicap, though there is some weak evidence that larger absolute starts are associated with slightly larger standard deviations.
For season 2009, the story's a little different. The overall average is +8.4 points which, the asterisks tell us, is statistically significantly different from zero at the 5% level. The standard deviations are much smaller and, if anything, larger absolute margins seem to be associated with smaller standard deviations.
Combining all the seasons, the aberrations of 2009 are mostly washed out and we find an average Home HAM of just +1.6 points.
Next, consider Favourite HAMs, the data for which appears below:
The first thing to note about this table is the fact that none of the Favourite HAMs are significantly different from zero.
Overall, across seasons 2006 to 2008 the average Favourite HAM is just 0.1 point; in 2009 it's just -3.7 points.
In general there appears to be no systematic relationship between the start given by favourites and the standard deviation of the resulting Favourite HAMs.
Summarising:
- Across seasons 2006 to 2009, Home HAMs and Favourite HAMs average around zero, as we hoped
- With a few notable exceptions, mainly for Home HAMs in 2009, the average is also around zero if we condition on either the handicap given by the Home Team (looking at Home HAMs) or that given by the Favourite Team (looking at Favourite HAMs).
Okay then, are Home HAMs and Favourite HAMs normally distributed?
Here's a histogram of Home HAMs:
And here's a histogram of Favourite HAMs:
There's nothing in either of those that argues strongly for the negative.
More formally, Shapiro-Wilks tests fail to reject the null hypothesis that both distributions are Normal.
Using this fact, I've drawn up a couple of tables that compare the observed frequency of various results with what we'd expect if the generating distributions were Normal.
Here's the one for Home HAMs:
There is a slight over-prediction of negative Home HAMs and a corresponding under-prediction of positive Home HAMs but, overall, the fit is good and the appropriate Chi-Squared test of Goodness of Fit is passed.
And, lastly, here's the one for Home Favourites:
In this case the fit is even better.
We conclude then that it seems reasonable to treat Home HAMs as being normally distributed with zero mean and a standard deviation of 37.7 points and to treat Favourite HAMs as being normally distributed with zero mean and, curiously, the same standard deviation. I should point out for any lurking pedant that I realise neither Home HAMs nor Favourite HAMs can strictly follow a normal distribution since Home HAMs and Favourite HAMs take on only discrete values. The issue really is: practically, how good is the approximation?
This conclusion of normality has important implications for detecting possible imbalances between the line and head-to-head markets for the same game. But, for now, enough.
Waiting on Line
/Hmmm. (Just how many ms are there in that word?)
It's Tuesday evening around 7pm and there's still no Line market up on TAB Sportsbet. In the normal course this market would go up at noon on Monday, and that's when the first match is on Friday night. So, this week the first game is 24 hours earlier than normal and the Line market looks as though it'll be delayed by 48 hours, perhaps more.
Curiouser still is the fact that the Head-to-Head market has been up since early March (at least) and there's an historical and strong mathematical relationship between Head-to-Head prices and the Line market, as the following chart shows.
The dark line overlaid on the chart fits the empirical data very well. As you can see, the R-squared is 0.944, which is an R-squared I'd be proud to present to any client.
Using the fitted equation gives the following table of Favourite's Price and Predicted Points Start:
Anyway, back to waiting for the TAB to set the terms of our engagement for the weekend ...
Is There a Favourite-Longshot Bias in AFL Wagering?
/The other night I was chatting with a few MAFL Investors and the topic of the Favourite-Longshot bias - and whether or not it exists in TAB AFL betting - came up. Such a bias is said to exist if punters tend to do better wagering on favourites than they do wagering on longshots.
The bias has been found in a number of wagering markets, among them Major League Baseball, horse racing in the US and the UK, and even greyhound racing. In its most extreme form, so mispriced do favourites tend to be that punters can actually make money over the long haul by wagering on them. I suspect that what prevents most punters from exploiting this situation - if they're aware of it - is the glacial rate at which profits accrue unless large amounts are wagered. Wagering $1,000 on a contest with the prospect of losing it all in the event of an upset or, instead, of winning just $100 if the contest finishes as expected seems, for most punters, like a lousy way to spend a Sunday afternoon.
Anyway, I thought I'd analyse the data that I've collected over the previous 3 seasons to see if I can find any evidence of the bias. The analysis is summarised in the table below.
Clearly such a bias does exist based on my data and on my analysis, in which I've treated teams priced at $1.90 or less as favourites and those priced at $5.01 or more as longshots. Regrettably, the bias is not so pronounced that level-stake wagering on favourites becomes profitable, but it is sufficient to make such wagering far less unprofitable than wagering on longshots.
In fact, wagering on favourites - and narrow underdogs too - would be profitable but for the bookie's margin that's built into team prices, which we can see has averaged 7.65% across the last three seasons. Adjusting for that, assuming that the 7.65% margin is applied to favourites and underdogs in equal measure, wagering on teams priced under $2.50 would produce a profit of around 1-1.5%.
In the table above I've had to make some fairly arbitrary decisions about the price ranges to use, which inevitably smooths out some of the bumps that exist in the returns for certain, narrower price ranges. For example, level-stake wagering on teams priced in the range $3.41 to $3.75 would have been profitable over the last three years. Had you the prescience to follow this strategy you'd have made 32 bets and netted a profit of 9 units, which is just over 28%.
A more active though less profitable strategy would have been to level-stake wager on all teams priced in the $2.41 to $3.20 price range, which would have led you to make 148 wagers and pocket a 3.2 unit or 2.2% profit.
Alternatively, had you hired a less well-credentialled clairvoyant and as a consequence instead level-stake wagered on all the teams priced in the $1.81 to $2.30 range - a strategy that suffers in part from requiring you to bet on both teams in some games and so guarantee a loss - you'd have made 222 bets and lost 29.6 units, which is a little over a 13% loss.
Regardless, if there is a Favourite-Longshot bias, what does it mean for MAFL?
In practical terms all it means is that a strategy of wagering on every longshot would be painfully unprofitable, as last year's Heritage Fund Investors can attest. That’s not to say that there's never value in underdog wagering, just that there isn’t consistent value in doing so. What MAFL aims to do is detect and exploit any value – whether it resides in favourites or in longshots.
What MAFL also seeks to do is match the size of its bet to the magnitude of its assessed advantage. That, though, is a topic for another day.